![\begin{multline}
\left( \frac{\partial}{\partial t}+L_1^0 \right)P(\mathbf{r}_1,...
...athbf{r}_1,\mathbf{v}_1,t)P(\mathbf{r}_1,\mathbf{v}_2,t) \right ]
\end{multline}](img674.png)
The Homogeneous Cooling State can be characterized at the kinetic or
at the hydrodynamics level. We expose both the characterizations.
Kinetics of the Homogeneous Cooling State
We rewrite here the Boltzmann Equation for a 3D cooling granular gas [42,210] (see paragraph 2.2.8):
The kinetic definition of Homogeneous Cooling State is given by the scaling ansatz for the state distribution function:
where
![]() and
and ![]() is the thermal
velocity defined by
is the thermal
velocity defined by
![]() with
with ![]() the temperature
(defined for example in Eq. (2.116)). If the Eq. (5.2)
is inserted in the Boltzmann Equation
(5.1), an equation for the
temperature is obtained:
the temperature
(defined for example in Eq. (2.116)). If the Eq. (5.2)
is inserted in the Boltzmann Equation
(5.1), an equation for the
temperature is obtained:
where
![]() is the surface area of a
is the surface area of a
![]() -dimensional unit sphere,
-dimensional unit sphere, ![]() is the time dependent collision
frequency, while
is the time dependent collision
frequency, while ![]() is the time independent cooling rate. These
last two functions are defined by:
is the time independent cooling rate. These
last two functions are defined by:
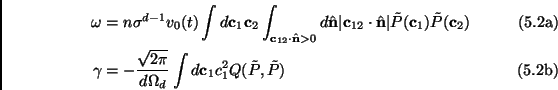 |
and can be approximated, using the Maxwellian approximation
![]() , by
, by ![]() and
and ![]() respectively, given by:
respectively, given by:
The solution of the temperature equation (5.3) reads:
where
![]() is the mean free time at the initial temperature
is the mean free time at the initial temperature ![]() and
and
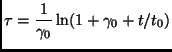 |
(5.6) |
is the cumulated collision number obtained from the definition
![]() . Eq. (5.6) is known as
Haff's law [103] and has been discussed in paragraph 2.4.1.
. Eq. (5.6) is known as
Haff's law [103] and has been discussed in paragraph 2.4.1.
Hydrodynamics of the Homogeneous Cooling State
The hydrodynamics for the Inelastic Hard Spheres model [39,214] is described by the following equations (see section 2.4):
These equations are generally closed with constitutive relations obtained by means of expansions of kinetic equations. The results presented in this section have been obtained by van Noije, Ernst, Brito and Orza [212] and are based on the following (Navier-Stokes order) approximations:
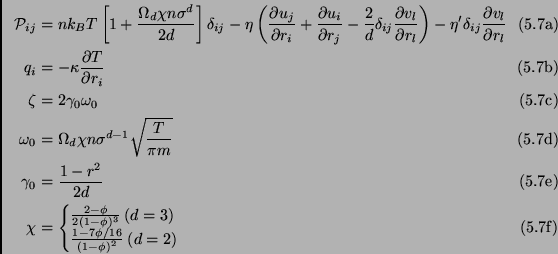 |
with ![]() ,
, ![]() and
and ![]() the shear viscosity, bulk viscosity
and heat conductivity respectively. The expression for the pressure
correction
the shear viscosity, bulk viscosity
and heat conductivity respectively. The expression for the pressure
correction
![]() and for the collision frequency correction
(which both contains the pair distribution function of hard spheres or
disks at contact
and for the collision frequency correction
(which both contains the pair distribution function of hard spheres or
disks at contact
![]() ) are obtained from the Enskog
theory (see paragraph 2.2.6), with
) are obtained from the Enskog
theory (see paragraph 2.2.6), with ![]() the volume fraction (
the volume fraction (
![]() if
if
![]() or
or
![]() if
if ![]() ).
).
The equations (5.8) are solved by the following homogeneous solution:
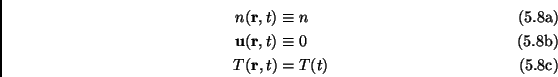 |
where ![]() is the same as in Eq. (5.6).
is the same as in Eq. (5.6).
An expansion of the kinetic equation in the inelasticity parameter
![]() has shown [210,198] that
has shown [210,198] that
![]() and
and ![]() are well approximated by
are well approximated by ![]() and
and
![]() (their Maxwellian counterparts) for almost all values of
(their Maxwellian counterparts) for almost all values of
![]() .
.