Linear stability analysis

A granular gas prepared with a homogeneous density and no macroscopic flow, at a given temperature $T(0)$, reaches the Homogeneous Cooling State in a few free times $t_0$. To study the behavior of small (macroscopic, i.e. for wave vectors of low magnitude $k \ll \min\{2\pi/l_0,2\pi/\sigma\}$) fluctuations around this state, a linear stability study of hydrodynamics equations (5.8) has been performed by several authors (Goldhirsch and Zanetti [96], Deltour and Barrat [73], van Noije et al. [214]). We follow the detailed discussion of [212], reviewing their result for the linearized hydrodynamics of rescaled fields. The rescaled fluctuation fields are defined as

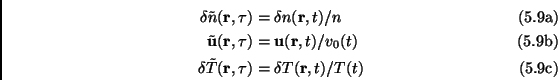Their Fourier transforms are given by $\delta \tilde{a}(\mathbf{k},\tau)=\int d\mathbf{r} \exp (-i\mathbf{k} \cdot \mathbf{r}) \delta \tilde{a}(\mathbf{r},\tau)$, where $a$ is one of $(n,\mathbf{u},T)$.

The vector $\tilde{\mathbf{u}}(\mathbf{k},\tau)$ can be decomposed in $(d-1)$ vectors perpendicular to $\mathbf{k}$, called indistinctly $\tilde{\mathbf{u}}_\perp$, and one vector parallel to $\mathbf{k}$, called $\tilde{\mathbf{u}}_\parallel$.

The linearized hydrodynamics for these fluctuations is given (in Fourier space) by the following equation:

$$\frac{\partial}{\partial \tau}\delta \tilde{\mathbf{a}}(\mathbf{k},\tau)=\tilde{\mathcal{M}}(\mathbf{k})\delta\tilde{a}(\mathbf{k},\tau)$$ (5.11)

where

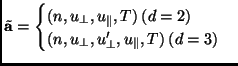$$\tilde{\mathbf{a}} = \begin{cases}(n, u_\perp, u_\parallel, T) \: (d=2) \ (n, u_\perp, u_\perp', u_\parallel, T) \: (d=3) \end{cases}$$ (5.12)

The matrix $\tilde{\mathcal{M}}$ is given (in $d=2$) by:

$$\tilde{\mathcal{M}}= \begin{pmatrix}0 & 0 & -ikl_0 & 0 \ 0 & \g... ...& 0 & -ikl_0\left(\frac{2p}{dnT}\right) & -\gamma_0(1+k^2\xi_T^2) \end{pmatrix}$$ (5.13)

Here we have introduced the correlation lengths $\xi_\perp$, $\xi_\parallel$ and $\xi_T$ that depend on the transport coefficients (shear and bulk viscosity and heat conductivity), on the isothermal compressibility $\chi_T=(\partial n/\partial p)_T/n$ and on the pair distribution function $g(n)$ already mentioned. We refer to [212] for detailed calculations of these correlation lengths.

We report in Fig. fig_disp_rel a plot published in several articles from van Noije and co-workers [176], that displays the linear dispersion relations, i.e. the exponential growth rates of the modes as functions of the wave number.

Figure: Growth rates $\zeta _\lambda /\gamma _0$ for shear ( $\lambda =\perp$), heat ($\lambda =H$) and sound ( $\lambda =\pm$) modes versus $k\sigma$ for inelastic hard disks with $r=0.9$ at a packing fraction $\phi =0.4$. The dashed line indicate the imaginary parts of the sound modes that vanish for $k \ll \gamma _0/l_0$. (From Orza et al. [176])

Several facts must be noted. The first is that (in this linear analysis) the evolution of fluctuations of normal velocity components (shear modes, $\tilde{\mathbf{u}}_\perp$) are not coupled with any other fluctuating component. At the same time, all the other components are coupled together. The study of eigenvalues and eigenvectors confirms the fact that the shear modes are not coupled with the other modes. The eigenvectors of the matrix define, beyond the shear modes, three other modes: one heat mode and two sound modes, denoted in the following with the subscripts $H$ and $+$ or $-$ respectively. The associated eigenvalues are $\zeta_\perp(k)$, $\zeta_H(k)$, $\zeta_+(k)$ and $\zeta_-(k)$. It is immediate to see that $\zeta_\perp(k)=\gamma_0(1-k^2\xi_\perp^2)$. At low values of $k$ (in the dissipative range defined below) also the heat mode is ``pure'', as it is given by the longitudinal velocity mode $\tilde{\mathbf{u}}_\parallel$ only, with eigenvalue $\zeta_H(k) \simeq \gamma_0(1-\xi_\parallel^2k^2)$; in this range the sound modes are combination of density and temperature fluctuations.

The most important result of this analysis is that $\zeta_\perp(k)$ and $\zeta_H(k)$ are positive below the threshold values $k_\perp^*=1/\xi_\perp\sim \sqrt{\epsilon}$ and $k_H^* \simeq 1/\xi_\parallel\sim \epsilon$ respectively, indicating two linearly unstable modes with exponential (in $\tau$) growth rates.

The shear and heat instabilities are well separated at low inelasticity, as $k_\perp^* \sim \sqrt{\epsilon}$ while $k_H^* \sim \epsilon$, so that $k_\perp^* \gg k_H^*$. It is also important to note that the linear total size $L$ of the system can suppress the various instability, as the minimum wave number $k_{min}=2\pi/L$ can be larger than $k_H^*$ or even than $k_\perp^*$.

Moreover, the study of the eigenvalues of the linear stability matrix, shows that several regimes in the $k$-space are present:

• for $2\pi/L \ll k \ll \gamma_0/l_0$ (dissipative range), all the eigenvalues are real, so that propagating modes are absent;

• for $\gamma_0/l_0 \ll k \ll \sqrt{\gamma_0}/l_0$ (standard range), the eigenvalues corresponding to sound modes are complex conjugates, so that the sound modes propagate;

• for $\sqrt{\gamma_0}/l_0 \ll k \ll \min\{2\pi/l_0,2\pi/\sigma\}$ (elastic range) the heat conduction become dominant; in this range the dispersion relations resemble those of an elastic fluid.

The above picture, of course, requires the scale separation $\gamma_0 \ll \sqrt{\gamma_0}$ (valid at low inelasticity).