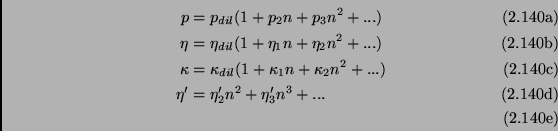 |
If the Boltzmann-Enskog equation is used as the basic kinetic equation, the transport coefficients and the scalar pressure change. In principle the transport coefficients (and the scalar pressure) can be expanded in powers of the density [109]:
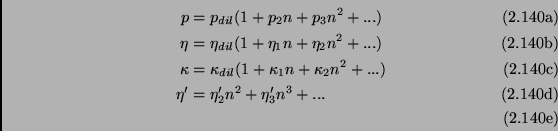 |
where
![]() is the scalar pressure in the dilute case
is the scalar pressure in the dilute case ![]() ,
,
![]() is the viscosity in the dilute
case,
is the viscosity in the dilute
case,
![]() is the heat conductivity
in the dilute case and
is the heat conductivity
in the dilute case and ![]() is the bulk viscosity which has
been neglected till now as it is of order
is the bulk viscosity which has
been neglected till now as it is of order ![]() . The bulk
viscosity modifies the expression for the stress tensor, introduced in
Eq. (2.144) i.e.:
. The bulk
viscosity modifies the expression for the stress tensor, introduced in
Eq. (2.144) i.e.:
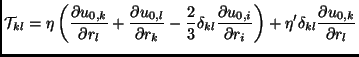 |
(2.142) |
The Enskog correction accounts for the appearance of position
correlations at high densities: these correlations render the high ![]() order terms important so that they must be included in analytical
expressions.
order terms important so that they must be included in analytical
expressions.
In ![]() the corrections are the followings:
the corrections are the followings:
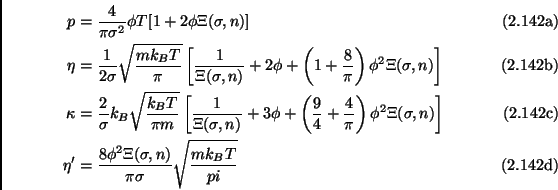 |
where ![]() is the solid fraction (see paragraph 2.2.6).
is the solid fraction (see paragraph 2.2.6).